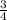Question

HELP PLZZ ASAP !! a. The value of x is 6 , what is the value of y?

Answer 1

a). y = 4.5

b) Scale factor =
`(3)/(4)`

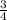
Explanation:

a). Since, polygon Q is the scaled copy of polygon P

Both the figures will be similar.

Therefore, their corresponding sides will be proportional.

`(x)/(y) =(4)/(3)`

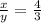
If x = 6,

`(6)/(y) =(4)/(3)`

y =
`(3* 6)/(4)`

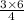
y = 4.5

b). Since, polygon Q is the scaled copy of polygon P,

Polygon Q will be the image of preimage polygon P.

Scale factor =
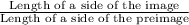
`\frac{\text{Length of a side of the image}}{\text{Length of a side of the preimage}}`

=
`(3)/(4)`