We know for the problem that Arnold drove 400 miles during his business trip, and the cost of each mile is $0.50, so the total cost of the miles the company will reimburse is:
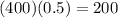
$.
Part A. We now know that the total cost of the miles the company have to pay to Arnold is $200. We also know the company pays him $40 a day for food and lodging. So let

represent the number of days of Arnold's business trip:
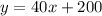
where

is the amount the company will reimburse Arnold after

days.

is the number of days.
Part B. We know that the amount the company will reimburse Arnold is $2600, so

. Lets replace that value in our equation and solve for

to find the number of days:
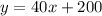

The first thing we are going to do to solve our equation is subtract 200 to both sides using the subtraction property of equality:

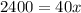
Next, we are going to divide both sides of the equation by 40, using the
division property of equality, to find the value of
 :
:
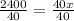
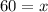
Finally, we can use the reflexive property of equality to get:
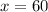
Part C. We can conclude that Arnold spend 60 days in his business trip.