Answer:
The feasible region is bounded by the corner points:
- (0, 0)
- (0, 8)
- (8, 4)
- (10, 0)
Explanation:
Given constraints:
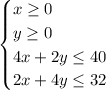
Rewrite the third and fourth inequalities to isolate y:
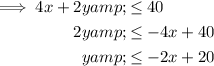
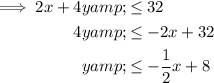
When graphing inequalities:
- < or > : dashed line.
- ≤ or ≥ : solid line.
- < or ≤ : shade under the line.
- > or ≥ : shade above the line.
Therefore:
- Draw the line x = 0 and shade above it (to the right).
- Draw the line y = 0 and shade above it.
- Draw the line y = -2x + 20 and shade below it.
- Draw the line y = -¹/₂x + 8 and shade below it.
The feasible region is the set of all possible values of the variables which satisfy the constraints.
Therefore, the feasible region is bounded by the corner points:
- (0, 0)
- (0, 8)
- (8, 4)
- (10, 0)