Answer:
Option 1. is correct
Explanation:
To find: the coordinates for point A
Solution:
From the graph, we can see coordinates of A =
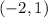
To find: Option which is not appropriate .
Solution:
For two points
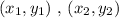 , distance is given by
, distance is given by

For AB,
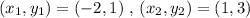

For BC,
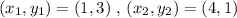
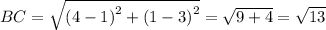
For AC,
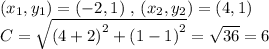
So, AB = BC i.e, two sides are equal . Therefore, the given triangle can not be right equilateral as in equilateral triangle , all sides are equal .
Option 1. is correct