Question

The product of 0.1400 × 6.02 × 1023 will have how many significant figures?

Answer 1

3 significant figures.

0.1400 has 4 sig figs, 6.02 has 3, and 1023 has 4. when calculating significant figures for a multiplication or division equation, the product or quotient will have the same amount of significant figures as the number with the least amount of significant figures (in this case: 6.02).

Answer 2

3

Explanation:

We are given that an expression

`0.1400* 6.02* 10^(23)`

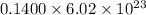
We have to find the number of significant figures in the product .

In 0.1400 , there are 4 significant figures

In
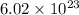`6.02* 10^(23)`, there are three significant figures

`0.1400* 6.02* 10^(23)`

`0.8428* 10^(23)`

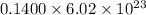
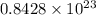
In multiplication, we have to precise the final answer to least significant figures.

Therefore, the final answer=
`0.843* 10^(23)`

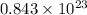
Hence, three significant figures in the product.