Answer: The co-ordinates of the image quadrilateral areA'(-3, -1), B'(-5, -4), C'(-7, -2) and D'(-4, 0). The length of the segments AD and A'D' are both √2 units.
Step-by-step explanation: We are given to list the co-ordinates of the image after a rotation of the figure below of 180° about the origin.
From the figure, we note that
the co-ordinates of the vertices of quadrilateral ABCD are A(3, 1), B(5, 4), C(7, 2) and D(4, 0).
We know that
the co-ordinates of the image of a point (x, y) after rotation of 180° about the origin is given by (-x, -y).
Therefore, after rotating the quadrilateral ABCD through an angle of 180° about the origin, then the co-ordinates of the image A'B'C'D' are
A(3, 1) ⇒ A'(-3, -1),
B(5, 4) ⇒ B'(-5, -4),
C(7, 2) ⇒ C'(-7, -2)
and
D(4, 0) ⇒ D'(-4, 0).
And, the lengths of the line segments AD and A'D' can be calculated using distance formula as follows :
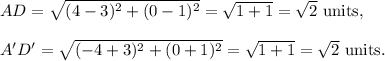
Thus, the co-ordinates of the image quadrilateral are A'(-3, -1), B'(-5, -4), C'(-7, -2) and D'(-4, 0). The length of the segments AD and A'D' are both √2 units.