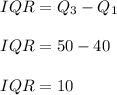Question

What is the interquartile range of the data set? Enter the answer in the box. Key: 1|5 means 15

Answer 1

The interquartile range(IQR) of the data set is:

10

Explanation:

The data points according to the stem and leaf plot are as follows:

28 40 43 43 45 50 50

We know that the median of the data is the central tendency of the data and always lie in the middle of the data.

Here looking at the data we have:

Median= 43

Similarly the lower set of data is:

28 40 43

Hence, the first quartile or the lower quartile i.e.
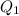`Q_1` is the median of the lower set of data.

Hence,

`Q_1=40`

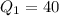
Similarly, the upper set of data is:

45 50 50

Hence, the upper quartile or the third quartile i.e.
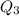`Q_3` is the median of the upper set of data.

Hence,

`Q_3=50`

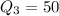
The interquartile range(IQR) is calculated as:

`IQR=Q_3-Q_1\\\\IQR=50-40\\\\IQR=10`

Answer 2

First of all we have to arrange the data in ascending order as shown below:

28, 40, 43, 43, 45, 50, 50

Total number of values = 7

Since the number of values is odd, the median will be the middle value i.e. 4th value which is 43. Median divides the data in two halves:

1st Half = 28, 40, 43
2nd Half = 45, 50, 50

Q1 or the First Quartile is the middle value of the lower or 1st half which is 40.
Q3 or the Third Quartile is the middle value of the upper or second half, which is 50.

IQR or the Inter Quartile Range is the difference of Q3 and Q1.

So, IQR= Q3 – Q1 = 50 – 40 = 10

Thus, IQR for the given data is 10