Question

PLEASE HELP ME ANSWER THIS QUESTION?

Answer 1

The proportion of radius will be the same with the proportion of the height. Make a comparison

`\cfrac{h_(1)}{h_(2)}=\cfrac{r_(1)}{r_(2)}`

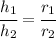
Input the numbers

`\cfrac{h_(1)}{h_(2)}=\cfrac{40}{16}`

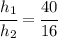
simplify the fraction, divide the numerator and denominator by 8

`\cfrac{h_(1)}{h_(2)}=\cfrac{5}{2}`

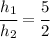
do cross multiplication
2h₁ = 5h₂
h₂ =
`(2)/(5)h_(1)`

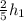
General formula to find the volume
1/3 × π × r² × h = volume
In the case of the first cone
1/3 × π × 40² × h₁ = 1,875
1/3 × π × 1,600 × h₁ = 1,875
1/3 × π × h₁ =
`(1,875)/(1,600)`
save it, we'll use this expression later

In the case of the second cone
We know that h₂ =
`(2)/(5)h_(1)`
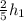
So the expression will be
1/3 × π × r₂² × h₂ = v₂
1/3 × π × 16² × h₂ = v₂
1/3 × π × 256 ×
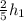
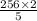
`(2)/(5)h_(1)` = v₂
1/3 × π × h₁ ×
`(256 * 2)/(5)` = v₂
1/3 × π × h₁ ×
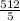
`(512)/(5)` = v₂

Use the expression we save, substitute 1/3 × π × h₁ with
`(1,875)/(1,600)`

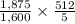
`(1,875)/(1,600) *(512)/(5)` = v₂
120 = v₂

The volume of the second cone is 120 cm³