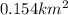Question

A rectangular field is 240m wide. The width of the field is 3/8 the length. What is the area of the field in square kilometers rounded to the nearest thousandth?

Answer 1

this means you should do 240*3/8 which is 90. so 90 times 240=21600
the answer is 216000km^2

Answer 2

Let the length of the rectangular field be x.

Given, the width of the field is 3/8 of the length.

So width =
`((3)/(8) )x` =
`(3x)/(8)`

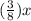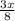
Given, the width of the rectangular field = 240m

So we can write the equation as,

`(3x)/(8) = 240`

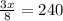
First we have to move 8 to the right side by multiplying 8 to both sides.

`((3x)/(8) )(8) = (240)(8)`

`3x = (240)(8)`

`3x = 1920`

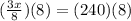
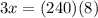
We can get x, by moving 3 to the right side by dividing 3 to both sides.

`(3x)/(3) = (1920)/(3)`

`x = (1920)/(3)`

`x = 640`

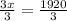
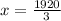
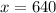
So we have got the length of the rectangular field = 640m = 0.64 km.

width of the field = 240m = 0.24 km.

We know the formula to find the area of the rectangle =
`(l)(w)`

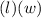
where,
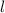`l` = length and
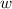`w` = width

By plugging in the values in the formula we will get,

Area =
`(0.64)(0.24)`
`km^2`

=
`0.1536 km^2` =
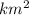
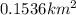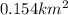`0.154 km^2` ( Approximately taken to the nearest thousandth)

So we have got the required answer.

The area of the rectangular field =
`0.154 km^2`