Answer:

The graph of the solution is attached.
Explanation:
Given inequality:
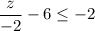
Add 6 to both sides:

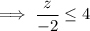
Multiply both sides by -2 (remembering to reverse the inequality sign as we are multiplying by a negative number):

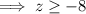

To graph the solution:
- Place a closed circle at -8 as "≥" indicates that -8 is part of the boundary.
- Shade to the right of the closed circle as "≥" means "greater than or equal to".
- Place an arrow (pointing to the right) at the end of the shading to indicate it continues without an end in that direction.