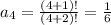Question

Determine the first four terms of the sequence in which the nth term is

Answer 1

The correct answer option is:
`(1)/(3) ,(1)/(4) ,(1)/(5) ,(1)/(6)`.

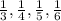Explanation:

We know that the
`nth` term
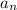`a_n` for an arithmetic sequence is given by:

`a_n=((n+1)!)/((n+2)!)`

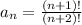
where
`n` is the number of the position of the term.

We are supposed to find the first four terms of the sequence so we will substitute the values of
`n` from 1 to 4 in the given formula to get:

1st term:

`a_1=((1+1)!)/((1+2)!)=(1)/(3)`

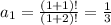
2nd term:

`a_2=((2+1)!)/((2+2)!)=(1)/(4)`

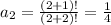
3rd term:

`a_3=((3+1)!)/((3+2)!)=(1)/(5)`

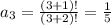
4th term:

`a_4=((4+1)!)/((4+2)!)=(1)/(6)`