Answer : The maximum number of grams of
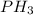 formed is, 8.955 g
formed is, 8.955 g
Solution : Given,
Mass of phosphorous = 8.2 g
Mass of hydrogen = 4 g
Molar mass of
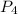 = 123.6 g/mole
= 123.6 g/mole
Molar mass of
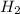 = 2.016 g/mole
= 2.016 g/mole
Molar mass of
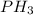 = 33.924 g/mole
= 33.924 g/mole
The balanced chemical reaction is,
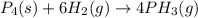
First we have to calculate the moles
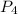 and
and
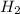
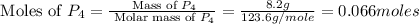
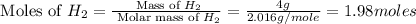
From the reaction, we conclude that
1 mole of
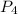 react with 6 moles of
react with 6 moles of
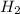
0.066 moles of
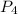 react with
react with
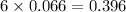 moles of
moles of
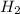
That means the
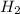 is in excess amount and
is in excess amount and
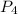 is in limited amount.
is in limited amount.
Now we have to calculate the moles of
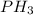 .
.
As, 1 mole of
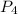 react to give 4 moles of
react to give 4 moles of
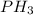
So, 0.066 moles of
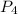 react to give
react to give
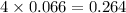 moles of
moles of
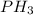
Now we have to calculate the mass of
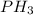

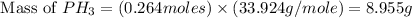
Therefore, the maximum number of grams of
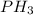 formed is, 8.955 g
formed is, 8.955 g