Answer:
C. Playing soccer and basketball are not independent since P(soccer|basketball) ≠ P(soccer) and P(basketball|soccer) ≠ P(basketball) .
Explanation:
We have the data of athletic people that played soccer and basketball.
Let, P(A) = probability of people who played soccer
P(B) = probability of people who played basketball
P(A∩B) = probability of people who played both soccer and basketball.
We will now find the probability of soccer | basketball
i.e.
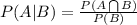
i.e.
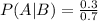 = i.e.
= i.e.

As,
 ≠
≠
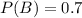
So, options A, B and D are discarded.
Moreover, i.e.
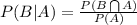
i.e.
 = i.e.
= i.e.
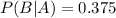
i.e.
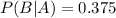 ≠
≠
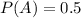
Hence, we get that playing soccer and basketball are not independent.