Question

Write the point-slope form of the equation of the line that passes through the points (6, 6) and (-6, 1). Include your work in your final answer. Type your answer in the box provided or use the upload option to submit your solution.

Answer 1

3/4(6,-1)

Explanation:

(6,6)*-(-6,1)

/by answer

Answer 2

`y = (5)/(12)x + (21)/(6)`

Explanation:

We are given the following information in the question.

The line passes through the point (6,6) and (-6,1).

We have to find the point slope form of the equation.

The point slope form of equation is given by:

`y-y_1 = m(x-x_1)\\\text{where}\\m = \displaystyle(y_2-y_1)/(x_2-x_1)\\\\\text{Putting}\\(6,6) = (x_1,y_1), (-6,1) = (x_2,y_2)\\\\y - 6 = (1-6)/(-6-6)(x - 6)\\\\y-6 = (-5)/(-12)(x-6)\\\\12(y-6) = 5(x-6)\\12y - 72 = 5x - 30\\12y = 5x -30 + 72\\12y = 5x +42\\\\y = (5)/(12)x + (42)/(12)\\\\y = (5)/(12)x + (21)/(6)`

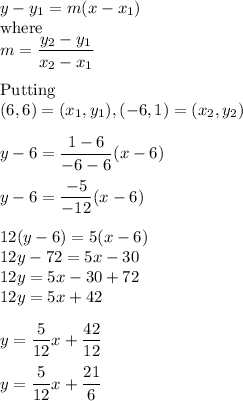
The above equation is the required slope intercept form of equation passing through the given points.