Looks like we have
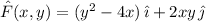
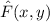 is conservative if we can find a scalar function
is conservative if we can find a scalar function
 such that
such that
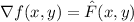 . For this to be the case, we would need to have
. For this to be the case, we would need to have

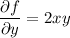
Take the first partial differential equation. If we integrate both sides with respect to
 , we'd get
, we'd get
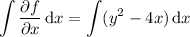

Differentiating both sides with respect to
 , we recover the other partial derivative and find
, we recover the other partial derivative and find

where
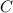 is an arbitrary constant. So we've found
is an arbitrary constant. So we've found
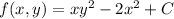
which means that
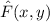 is conservative.
is conservative.