Question

Below is a proof showing that the sum of a rational number and an irrational number is an irrational number.

Answer 1

The statement that completes the proof is:

It is the sum of two rational numbers.

Explanation:

We will prove the statement by taking an assumption that:

The sum of a rational number a and an irrational number b is a rational number and is denoted by x.

i.e.

`a+b=x`

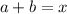
Now, it could also be written as:

`b=x+(-a)`

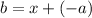
We know that if a is a rational number then (-a) is also a rational number.

Because the sign of the number changes not it's behavior.

Also, we know that the sum of two rational number's is always rational.

i.e.

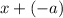`x+(-a)` will be a rational number.

i.e. b is also rational which will be a contradiction.

( Since it was given that b is an irrational number)

Answer 2

It is the sum of two rational numbers

Explanation:

Given that a is a rational number and b is an irrational number.

To prove that a+b is irrational.

Here contrapositive method is used to prove

If possible assume a+b is rational and equal to x

Then we have

a+b = x

b = x-a = x+(-a)

On right side we have sum of two rational numbers hence right side is rational.

But left side b is irrational thus a contradiction