Question

In △ABC, CH=33 ft.

What is the length of CX ?

Enter your answer in the box.


= ft

Answer 1

Hello there! Your answer is 22

Answer 2

CX = 11 ft.

Explanation:

The image is showing a triangle with its three medians. The point X represents the centroid of the triangle, because it's the intersection of all three medians.

There's a theorem about medians of a triangle that allow us to say that

`CX=(2)/(3)HC`

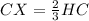
We already know that

`HC = CH = 33`

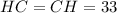
Replaing this value, we have

`CX=(2)/(3)33=2(11)=22`

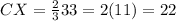
Then, by sum of segments, we have

`CH=CX+HX`

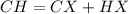
Replacing values, we have

`33=22+HX\\HX=33-22=11`

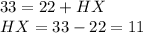
Therefore, CX measures 22 feet and HX measures 11 feet.