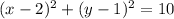Answer:
Option B is right.
Explanation:
We know that a circle is a locus of points keeping equal distance r from a fixed point called centre.
Here we have centre as (2,1)
Hence equation of the circle is given by
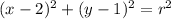
To find r, we can use the fact that (5,2) lies on this circle
Hence (5,2) satisfies this equatin.
So
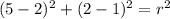
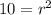
So equation of the circle is