Question

1. Write and equation in slope-intercept form of the line that passes through the given point and is parallel to the graph of the given equation.

(2,-2);y=-x-2

A)y=-2x
B)y=2x
C)y=1/2x
D)y=-x

2. Write and equation in slope-intercept form of the line that passes through the given point and is parallel to the graph of the given equation.
(2,-1);y=-3/2x+6

A)y=-3/2x+1
B)y=-3/2x-1
C)y=-3/2x+2
D)y=-3/2x+4

3. Write and equation in slope-intercept form of the line that passes through the given point and is parallel to the graph of the given equation.
(4,2);x=-3

A)y=2
B)y=2x+4
C)y=4x
D)x=4

4. Write and equation in slope-intercept form of the line that passes through the given point and is perpendicular to the graph of the given equation.
(-2,3);y=1/2x-1

A)y=1/2x+1
B)y=-2x-1
C)y=1/2x-1
D)y=-1/2x-1

5. Write and equation in slope-intercept form of the line that passes through the given point and is perpendicular to the graph of the given equation.
(5,0);y+1=2(x-3)

A)y=-1/2x+5
B)y=2x-5
C)y=1/2x-2
D)y=-1/2x+5/2

Answer 1

1) for two lines be parallel the slope must be the same , now the slope is -1, with the points given (2,-2) you can make the equation

y-y1=m(x-x1)

y-(-2)= -(x-2) ⇒y+2= -x+2⇒y=-x+2-2⇒y =-x ( option D )

2) for it equal than first

m= -3/2 and P(2,-1)

Y-Y1=M(X-X1) =

Y-(-1) = -3/2(X-2) ⇒Y+1 =-3/2X+3⇒Y= -3/2X+3-1⇒Y =-3/2X+2

4) For two lines be perpendicular the products of its slopes must be -1

m1*m2= -1, if m2 = 1/2 ⇒m1*1/2 = -1⇒m1= -2

now with the slope and the equation

y-y1=m(x-x1) ⇒y-3=-2[(x-(-2)]⇒y-3= -2x-4⇒y= -2x-4+3⇒y= -2x-1 ( 0ption b)

4) yo must set the equation to the form y = mx *b, y+1 = 2x-6 ⇒y=2x+6-1⇒y =2x+5

then m1*m2= -1⇒if m2 = 2⇒m1*2=-1⇒m1 = -1/2

now y-y1 =m(x-x1) ⇒y-0=-1/2(x-5)⇒y= -1/2x+5/2

Answer 2

1. The correct option is D.

2. The correct option is C.

3. The correct option is D.

4. The correct option is B.

5. The correct option is D.

Explanation:

The slope intercept form of a line is

`y=mx+b`

where, m is slope and b is y-intercept.

The slope of parallel lines are same.

(1)

The required line is parallel to the line y=-x-2 and passes through (2,-2). Slope of the line is -1.

The equation of required line is

`y-y_1=m(x-x_1)`

`y-(-2)=-1(x-2)`

`y+2=-x+2`

`y=-x`

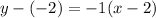
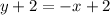
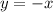
Therefore the correct option is D.

(2)

The required line is parallel to the line y=-3/2x+6 and passes through (2,-1). Slope of the line is -3/2.

The equation of required line is

`y-y_1=m(x-x_1)`

`y-(-1)=-(3)/(2)(x-2)`

`y+1=-(3)/(2)x+3`

`y=-(3)/(2)x+2`

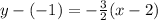
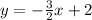
Therefore the correct option is C.

(3)

The required line is parallel to the line x=-3 and passes through (4,2). Slope of the line is infinite.

The equation of required line is

`y-y_1=m(x-x_1)`

`y-2=(1)/(0)(x-4)`

`0=x-4`

`x=4`

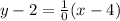
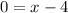
Therefore the correct option is D.

(4)

Product of slopes of perpendicular lines is -1.

The required line is perpendicular to the line y=1/2x-1 and passes through (-2,3). Slope of the required line is -2.

The equation of required line is

`y-y_1=m(x-x_1)`

`y-3=-2(x-(-2))`

`y-3=-2x-4`

`x=-2x-1`

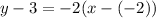
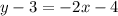
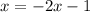
Therefore the correct option is B.

(5)

The required line is perpendicular to the line y+1=2(x-3) and passes through (5,0). Slope of the required line is -1/2.

The equation of required line is

`y-y_1=m(x-x_1)`

`y-0=-(1)/(2)(x-5)`

`y=-(1)/(2)(x)+(5)/(2)`

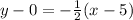
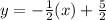
Therefore the correct option is D.