Question

Please select the best answer from the choices provided

Answer 1

The correct answer option is b. 3.

Explanation:

We are given an expression
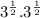`3^{(1)/(2) }.3^{(1)/(2) }` and we are supposed to simplify it.

We know that
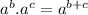`a^b.a^c=a^(b+c)` which means if the bases are same, then the exponents are added up.

So here, our bases are same i.e. 3 so we will add up the exponents to get:

`3^{(1)/(2)+(1)/(2) }`

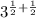
Taking LCM of the exponents to get:

`3^{(1+1)/(2) }\\\\3^(2)/(2)`

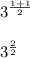
The fraction in the exponent gets cancelled so we are left with 3.

Answer 2

3^ (1/2) * 3^ (1/2)

add the exponents when the bases are the same

3^ (1/2+1/2)

3^ 1

3

Choice B