Question

Which of the following is not an equation of a simple, even polynomial function? (Select all to apply.)

y = | x |
y = x2
y = x3
y = -x2

Need Help.

Answer 1

Wouldn't it be y = | x |? I am not 100% positive but I'm pretty sure that you need to have a least one value. Again not 100% sure

Answer 2

`y=x^3`

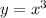
Explanation:

A function is an even polynomial function when f(-x) is equal to f(x)

Lets check each option, replace x with -x and see whether we get same y equation

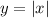`y=|x|`, Replace x with -x

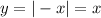`y=|-x|=x`, for any x value the value of y is positive always. So it is an even function

`y=x^2`

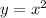
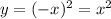`y=(-x)^2=x^2` same as the given equation . So it is an even function

`y=x^3`

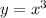
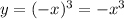`y=(-x)^3=-x^3` It is not same as the given equation . So it is not an even function

`y=-x^2`

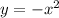
`y=-(-x)^2=-x^2` same as the given equation . So it is an even function