There are two ways to equate a straight line. We first have y=mx+b. Then, we have (y-y₁)=m(x-x₁). Both work fine and have similar variables, but the numbers are mixed around a bit. Your equation clearly shows the second form of equation. As our equation has x-x₁ on the right, we can notice that x+1 must mimic that, so x+1=(x-x₁). As x-(-1)=x+1, we can only assume that x is -1. Looking at the points given to us, y must be -2, so we have y-(-2)=y+2, so 2 fills in the leftmost open box. To find the slope, or m, we must take
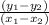 from points (x₁, y₁) and (x₂, y₂). It doesn't matter which point is (x₁, y₁) , but it matters that the y₁ corresponds to the x₁. Thus, we have our slope as
from points (x₁, y₁) and (x₂, y₂). It doesn't matter which point is (x₁, y₁) , but it matters that the y₁ corresponds to the x₁. Thus, we have our slope as

Feel free to ask further questions, and Happy Halloween!