Question

I was having trouble with this problem, and problems like it: A 3.2 kg pelican, with a 1.73 kg fish in its mouth, is flying 1.52 m/s at a height of 40 m when the fish wiggles free and fall back toward the ocean. How fast is the fish moving when it hits the water?

Answer 1

28.1 m/s

Step-by-step explanation:

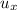`u_x` = Initial velocity of the fish = 1.52 m/s

y = Height of the bird = 40 m

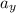`a_y` = Acceleration in y axis =
`9.81\ \text{m/s}^2`

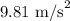
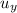`u_y` = Initial velocity in y axis = 0

`y=u_yt+(1)/(2)a_yt^2\\\Rightarrow 40=0+(1)/(2)* 9.81t^2\\\Rightarrow t=\sqrt{(40* 2)/(9.81)}\\\Rightarrow t=2.86\ \text{s}`

`v_y=u_y+a_yt\\\Rightarrow v_y=0+9.81* 2.86\\\Rightarrow v_y=28.057\ \text{m/s}`

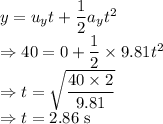
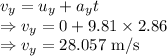
The final velocity in x direction will remain the same as the initial velocity as there is no acceleration in the x direction
`u_x=v_x=1.52\ \text{m/s}`

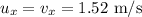
Resultant velocity is given by

`v=√(v_x^2+v_y^2)\\\Rightarrow v=√(1.52^2+28.057^2)\\\Rightarrow v=28.1\ \text{m/s}`

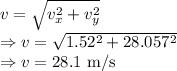
The fish is moving at a velocity of 28.1 m/s when it hits the water.