Question

15. Tim buys a new computer for his office for $1200. For tax purposes, he declares a linear depreciation (loss of value) of $200 per year. Let y be the declared value of the computer after x years. What is the slope of the line that models this depreciation?

Find the y-intercept of the line.

Write a linear equation in slope-intercept form to model the value of the computer over time.


Find the value of the computer after 4.5 years.

Answer 1

Slope is -200 and y-intercept is at (0, 1200)

The linear equation is:
`y=-200x+1200`

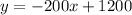
The value of the computer after 4.5 years will be $300.

Explanation:

Value of the new computer is $1200

For tax purposes, the linear depreciation (loss of value) is $200 per year.

So after
`x` years, total loss of value will be:
`\$200x`

If
`y` is the declared value of the computer after
`x` years, then the linear equation will be.......

`y=1200-200x\\ \\ \Rightarrow y=-200x+1200`

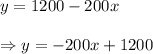
If we compare the above equation with slope-intercept form
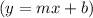`(y=mx+b)`, then we will get:
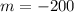`m=-200` and
`b=1200`

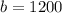
So, the slope of the line will be -200 and y-intercept will be at (0, 1200)

After 4.5 years means
`x=4.5`

So when
`x=4.5`,
`y=-200(4.5)+1200=-900+1200=300`

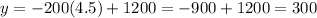
Thus, the value of the computer after 4.5 years will be $300.