Answer:
Option C
Explanation:
We are given that

We have to find that which pair of angles is not complementary
OD is perpendicular to FB
Therefore,
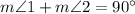
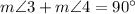
Substitute the values then we get
 (
(
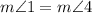 )
)
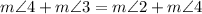
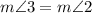
Substitute the value then we get
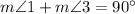
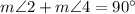
Complementary angles are that angles whose two angles sum is 90 degrees.
Hence, angle 2 and angle 3 are not complementary angles.
Option C is true.