Answer with explanation:
Given : There are 32 forwards and 80 guards in Leos basketball league.
Leo must include all players on a team and wants each team to have the same number of forwards and the same number of guards.
Then, the greatest number of teams possible= greatest common factor of 32 and 80
Prime factorization of 32 and 80:-
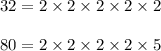
∴ GCF(32, 80)=

Number of guards in each team =
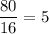
Hence, there are 5 guards in each team.