Answer: Fourth option is correct.
Explanation:
Since we have given that
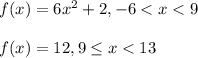
We need to find the value of f(x) when x = 6.
As we can see that x = 6 is lie in the interval -6<x<9.
So, f(x) would be
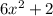
So, value of f(x) at x = 6 would be
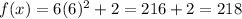
Hence, fourth option is correct.