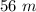Question

Um pequeno agricultor do interior do pará, ao medir seu terreno retangular, percebeu que suas dimensão estão na razão 5/7. Se a área do terreno é de 2240 m**, então sua maior dimensão em metros é de:

a)36
b)40
c)48
d) 56
e)62

Answer 1

The question in English

A small farmer in the interior of Par, when measuring his rectangular terrain, realized that his dimension is in reason 5/7. If the terrain area is 2240 m2, then its largest dimension in meters is

Let

x--------> the smallest dimension of the rectangular terrain

y--------> the largest dimension of the rectangular terrain

we know that

the area of the rectangular terrain is

`A=x*y`

`A=2,240\ m^(2)`

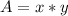
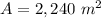
so

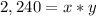`2,240=x*y` -------> equation A

`(x)/(y)=(5)/(7)`

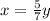`x=(5)/(7)y` -------> equation B

substitute the equation B in equation A

`2,240=[(5)/(7)y]*y\\\\5y^(2)= 15,680\\ \\y^(2)=3,136\\ \\y=56\ m`

therefore

the answer is the option D

`56\ m`