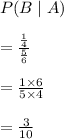Question

Two number cubes are rolled for two separate events: Event A is the event that the sum of numbers on both cubes is less than 10. Event B is the event that the sum of numbers on both cubes is a multiple of 3. Complete the conditional-probability formula for event B given that event A occurs first by writing A and B in the blanks: P_________( a0 | _________ a1) = P_______( a2 ∩ _____________a3) P(__________________ a4) If someone could please comment cant figure this question out

Answer 1

Answer:
`P(B\mid A)=(3)/(10)`

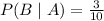
Explanation:

Since we have given that

A: getting the sum of number on both cubes is less than 10

B: getting the sum of numbers on both cubes is a multiple of 3

Conditional probability for event B given that A occurs first :

`P(B\mid A)=(P(A\cap B ))/(P(A))`

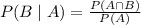
A ={(1,1)(1,2),(1,3),(1,4),(1,5),(1,6),(2,1),(2,2),(2,3),(2,4),(2,5),(2,6),(3,1),(3,2),(3,3),(3,4),(3,5),(3,6),(4,1),(4,2),(4,3),(4,4),(4,5),(5,1)(5,2),(5,3),(5,4),(6,1)(6,2)(6,3)}

B={(1,2),(2,1),(4,2)(2,4),(3,3),(5,4)(4,5),(3,6),(6,3)}

`P(A)=(30)/(36)\\\\=(5)/(6)`

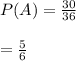
and

`P(B)=(9)/(36)=(1)/(4)`

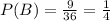
and

`P(A\cap B)=(9)/(36)\\\\=(1)/(4)`

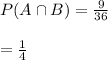
So using the formula,

`P(B\mid A)\\\\=((1)/(4))/((5)/(6))\\\\=(1* 6)/(5* 4)\\\\=(3)/(10)`