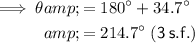Question

100 points! The picture has the question

Answer 1

4) do the x and y components

r = sqrt( (50)^2 + (34.6)^2 )

= 60.8

theta = tan-1 (34.6/50)

= 34.7 degrees

5) equal and opposite

r=60.8

theta =34.7 +180

=214.7 degrees

See the answer

Step-by-step explanation:

Answer 2

4. |R| = 60.8 N (3 s.f.)

θ = 34.7° (3 s.f.) relative to the x-axis

5. |F| = 60.8 N (3 s.f.)

θ = 214.7° (3 s.f.) relative to the x-axis

Step-by-step explanation:

`\textsf{Force}: \quad F=xi+yj`

`\textsf{Magnitude}: \quad |F|=√(x^2+y^2)`

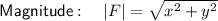
Given:

`|F_1|=30\;\sf N`

`|F_2|=40\;\sf N`

Using the given magnitudes and the force diagram:

`F_1=30i+0j`

`\begin{aligned}F_2&=(40 \cos 60^(\circ))i+(40 \sin60^(\circ))\\ &=20i+20√(3)j\end{aligned}`

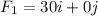
Question 4

The resultant force is the sum of all forces acting on an object.

To find the resultant force, add the corresponding components of the forces:

`\begin{aligned}\implies R& =(x_(F_1)+x_(F_2))i+(y_(F_1)+y_(F_2))j\\ &=(30+20)i+(0+20√(3))j\\& = 50i+20√(3)j\end{aligned}`

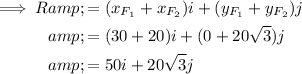
Therefore the magnitude of the resultant force is:

`\begin{aligned}\implies |R|&=\sqrt{50^2+(20√(3))^2\\& = √(2500+1200)\\& = √(3700)\\ & = √(100 \cdot 37)\\ & = √(100)√(37)\\ & = 10√(37)\\ & = 60.8\; \sf N\;(3\:s.f.)\end{aligned}`

The direction of the resultant force is:

`\begin{aligned}\implies \theta & = \tan^(-1)\left((y)/(x)\right)\\\theta & = \tan^(-1)\left((20√(3))/(50)\right)\\\theta & =34.71500395...^(\circ)\\\theta & =34.7^(\circ)\; \sf (3 \:s.f.)\end{aligned}`

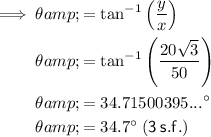
Question 5

An object is in equilibrium if the resultant force on it is zero.

Therefore, the magnitude of the equilibriant force is equal to the magnitude of the resultant force: 60.8 N (3 s.f.).

The direction of the equilibriant force is opposite to the resultant force, so its components will be:

`F=-50i-20√(3)j`

Therefore, it will be in Quadrant III.

So the direction relative to the x-axis will be the same direction as the resultant force plus 180°:

`\begin{aligned}\implies \theta &=180^(\circ)+34.7^(\circ)\\& = 214.7^(\circ)\; \sf (3 \:s.f.)\end{aligned}`