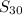Question

Need help again asap please! Find the sum of the first 30 terms of the sequence below an=3n+2

Answer 1

`a_n=3n+2\\a_(n+1)=3(n+1)+2=3n+3+2=3n+5\\\\a_(n+1)-a_n=(3n+5)-(3n+2)=3n+5-3n-2=3=const.\\\\\text{It's an arithmetic sequence where}\\a_1=3(1)+2=3+2=5\ and\ d=3\\\\\text{The formula of a sum of the first n terms of an arithmetic sequence:}\\\\S_n=(2a_1+(n-1)d)/(2)\cdot n\\\\\text{We have:}\\a_1=5,\ d=3\ \text{and}\ n=30\\\\\text{Substitute}\\\\S_(30)=(2(5)+(30-1)(3))/(2)\cdot30=(10+(29)(3))/(1)\cdot15=(10+87)(15)\\\\=(97)(15)=1455\\\\Answer:\ 1455`

Answer 2

`S_(30)` = 1455

generate a few terms of the sequence using
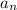`a_(n)` = 3n + 2

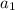`a_(1)` = ( 3 × 1) + 2 = 5

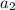`a_(2)` = (3 × 2) + 2 = 8

`a_(3)` = (3 × 3 ) + 2 = 11

`a_(4)` = (3 × 4 ) + 2 = 14

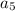`a_(5)` = ( 3 × 5 ) + 2 = 17

the terms are 5, 8, 11, 14, 17

these are the terms of an arithmetic sequence

sum to n terms is calculated using
`S_(n)` =
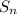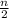`(n)/(2)` [ 2a + (n-1)d]

where a is the first term and d the common difference

d = 8 - 5 = 11 - 8 = 14 - 11 = 3 and
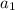`a_(1)` = 5

`S_(30)` =
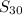`(30)/(2)` [( 2 × 5) + (29 × 3) ]

= 15( 10 + 87) = 15 × 97 = 1455