Answer:
17.58% probability that no student gets exactly 100 heads
Explanation:
We use the binomial probability distribution twice to solve this question.
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.
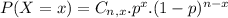
In which
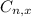 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.
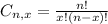
And p is the probability of X happening.
Probability of a single student getting 100 heads.
The coin is tossed 200 times, so
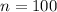
For each toss, 50% probability of getting heads, so
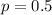
Then
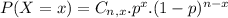
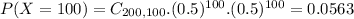
Approximately what is the chance that no student gets exactly 100 heads?
Each student has a 5.63% probability of getting exactly 100 heads, so
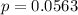
30 students, so

We have to find P(X = 0).
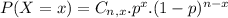
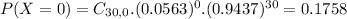
17.58% probability that no student gets exactly 100 heads