The absolute value of a number
 is the positive version of that number. So, if
is the positive version of that number. So, if
 is positive, its absolute value is
is positive, its absolute value is
 itself. If
itself. If
 is negative, its absolute value is
is negative, its absolute value is
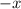 , so that it will be positive again.
, so that it will be positive again.
So, if the absolute value of a number is smaller than a certain quantity, i.e.
 it means that the positive version of
it means that the positive version of
 is less than
is less than
 .
.
So, if
 is positive, the inequality becomes
is positive, the inequality becomes
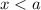 . If
. If
 is negative, the inequality becomes
is negative, the inequality becomes
 .
.
So, as a compound inequality, we have
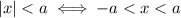
So, in your case, we have
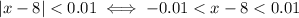
Add 8 to all sides in this inequality:
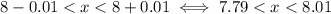
So, on a number line, you must higlight all numbers between 7.79 and 8.01, endpoints excluded.
Conceptually, this means that the bolt measurement should be exactly 8mm, but you can accept bolts that are 0.01 millimeters shorter of larger.