Question

Simplify 5p2/5p3

a) p/4
b) 3p3
c) 1/p
d) 6/7p3

Answer 1

To simplify the above expression, we can use the quotient rule.

`(x^a)/(x^b) =x^(a-b)`

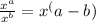
By using the above property we can subtract the exponents of the same base.

First step is to simplify the above expression, cancel out 5 from both numerator and denominator. Therefore,

`(5p^2)/(5p^3) =(p^2)/(p^3)`

=
`p^(2-3)`

=
`p^-1`

=
`(1)/(p)`

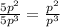
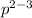
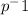
Since
`x^-1 = (1)/(x)`.

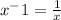So, the correct choice is c) 1/p.

I hope this helps you!