ANSWER
B. quadrilateral
Step-by-step explanation
To determine the type of quadrilateral we need to find the slope of each side.
Slope of the line connecting Z(0,3) and W(3,4).

Slope of the line connecting X(4,-1) and W(3,4).

Slope of the line connecting X(4,-1) and Y(0,-3).
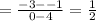
Slope of the line connecting Z(0,3) and Y(0,-3).

The slope of this line is not defined because it is a vertical line.
Since none of the slopes are equal, the quadrilateral can not be a trap-ezoid or a parallelogram or a rectangle.
Therefore the quadrilateral a WXYZ is just a quadrilateral.
The correct answer is B.