Question

The equation for line c can be written as y= – 3/7x+4. Perpendicular to line c is line d, which passes through the point (2,4). What is the equation of line d?

Answer 1

Answer:
`\text{y} = (7)/(3)\text{x}-(2)/(3)`

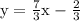
This is the same as writing y = (7/3)x - 2/3

=======================================================

Step-by-step explanation:

The given equation is in y = mx+b form

m = -3/7 = slope

b = 4 = y intercept

Flip the fraction for the slope to get -7/3, and flip the sign from negative to positive to get 7/3

The original slope is -3/7 and the perpendicular slope is 7/3. These two slopes multiply to -1.

We want the perpendicular line to go through
`(\text{x}_1,\text{y}_1) = (2,4)\\\\`

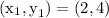
We'll use the point-slope form to get...

`\text{y} - \text{y}_1 = \text{m}(\text{x}-\text{x}_1)\\\\\text{y} - 4 = (7)/(3)(\text{x}-2)\\\\\text{y} - 4 = (7)/(3)\text{x}+(7)/(3)(-2)\\\\\text{y} - 4 = (7)/(3)\text{x}-(14)/(3)\\\\\text{y} = (7)/(3)\text{x}-(14)/(3)+ 4 \\\\\text{y} = (7)/(3)\text{x}-(14)/(3)+ (12)/(3) \\\\\text{y} = (7)/(3)\text{x}+(-14+12)/(3) \\\\\text{y} = (7)/(3)\text{x}-(2)/(3) \\\\`

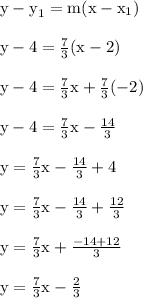
This equation has a slope of 7/3 and y intercept of -2/3.