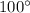Answer:
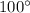
Explanation:
We are given that
AR=4
RC=4.5
BR=3
Let RD=x
Two chords are intersect to each other at R .
Product of AR and CR =product of BR and RD
Therefore,
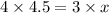
18=3x
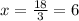
Point P is the center of circle.
BD=BR+RD=3+6=9 units
Radius=
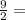 4.5 units
4.5 units
a.Circumference of given circle =

Circumference of given circle =
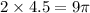 units
units
Area of circle=
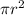
Area of circle=
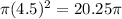
Area of circle=
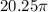 square units
square units
b.We are given that
Arc COD measure=
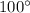
Arc BSA measure =
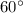
We have to find the measure of angle CRB
Measure of angle CRD is the average of arc COD and BSA
Therefore,Angle CRD=
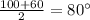
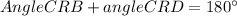 ( linear pair sum)
( linear pair sum)
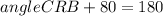
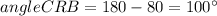
Hence, the measure of angle CRB=