Answer : The true statement is, Each text message costs 5 cents more than each anytime minute.
Step-by-step explanation :
Let the anytime minutes be, x and text messages be, y.
As we are given that a month he used 200 anytime minutes and 400 text messages, and paid $80.00. The equation will be:
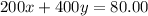 ...........(1)
...........(1)
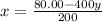 ............(A)
............(A)
And,
As we are given that a month he used 150 anytime minutes and 350 text messages, and paid $67.50. The equation will be:
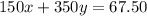 ...........(2)
...........(2)
Now solving these two equation by substitution method by putting equation A in equation 2, we get:
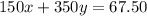
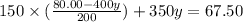
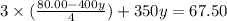



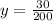

As we know that, $ 1 = 100 cents
So, $ 0.15 = 15 cents
y = 15 cents
Now put the value of 'y' in equation A, we get:
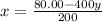
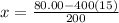

x = 10 cents
As per given statements we conclude that, Each text message costs 5 cents more than each anytime minute is the correct statements.
Proof :
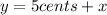
x = 10 cents

Thus, the true statement is, Each text message costs 5 cents more than each anytime minute.