The right answer is -36
This problem asks for the value of:
![6[4-9.4]](https://img.qammunity.org/2019/formulas/mathematics/high-school/cybvhdvn99sxnput8ylx9x0lxpuypqp0h5.png) .
.
So the function
![f(x)=[x]](https://img.qammunity.org/2019/formulas/mathematics/high-school/1nuebfzc3jgtlrod0vqrjj6ly7i6wk7da7.png) is called the gratest integer function.
is called the gratest integer function.
Functions whose graphs resemble sets of stairsteps are known as step functions. The most famous of the step functions is the greatest integer function, which is denoted by
![[x]](https://img.qammunity.org/2019/formulas/mathematics/high-school/ef3okl8gmwzykf5ipaga8zehy7wonakgwn.png) .
.
So, this function is defined as:
![f(x)=[x] \ the \ greatest \ integer \ less \ than \ or \ equal \ to \ x.](https://img.qammunity.org/2019/formulas/mathematics/high-school/71wyo80y4esauo18l6j6gx343535akuvby.png) .
.
The graph of the greatest integer function has the following characteristics:
1. The domain of the function is the set of all real numbers.
2. The range of the function is the set of all integers.
3. The graph has a
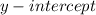 at
at
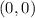 and
and
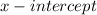 in the interval
in the interval
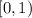
4. The graph is constant between each pair of consecutive integers.
5. The graph jumps vertically one unit at each integer value.
So, the value we are looking for is:
![f(x)=6[x] \\ \\ \therefore x=4-9.4=-5.4 \\ \\ \therefore f(-5.4)=6[-5.4]](https://img.qammunity.org/2019/formulas/mathematics/high-school/szlap1swv7pws05dm42ytu40b00doa75qz.png)
From the figure
![[-5.4]=-6](https://img.qammunity.org/2019/formulas/mathematics/high-school/cavvzl3wujszo81kuloin2ay16m4lsfcyc.png) , therefore:
, therefore:
![\boxed{6[4-9.4]=f(-5.4)=6(-6)=-36}](https://img.qammunity.org/2019/formulas/mathematics/high-school/7qpgvv5e19jibgquzurukl7efwkxgepe7i.png)