Question

Chad casts a shadow that is 14.3 feet long. The straight-line distance from the top of Chad’s head to the end of the shadow creates a 23° angle with the ground. How tall is Chad, to the nearest tenth of a foot?

Answer 1

Imagine right triangle with first leg to be the Chad length and second leg to be the Chad's shadow. Second leg has length 14.3 ft and since the straight-line distance from the top of Chad’s head to the end of the shadow creates a 23° angle with the ground, you could consider the trigonmetric function


`\tan 23^(\circ)= \frac{\text{Chad's length}}{\text{Chad's shadow length}}`,

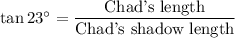then
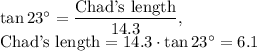`\tan 23^(\circ)= \frac{\text{Chad's length}}{14.3}, \\ \text{Chad's length}=14.3\cdot \tan 23^(\circ)=6.1`.