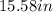To solve this problem you must apply the proccedure shown below:
By definition, an equilateral triangle has equal sides and all its internal angles are
 . If you divide it into two right triangles, you can apply the Pythagorean Theorem to calculate the height of the triangular base of the pyramid, as following:
. If you divide it into two right triangles, you can apply the Pythagorean Theorem to calculate the height of the triangular base of the pyramid, as following:
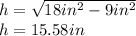
Therefore, as you can see, the answer is: