Question

Write parametric equations of the line with the equation 4x-6y=-12

Answer 1

To solve this problem you must apply the proccedure shown below:

1- You have the following equation given in the problem above:

`4x-6y=-12`

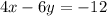
2- You can write as two different parametric equations:

A) - Solve for
`y`:

`4x-6y=-12\\6y=12+4x\\ y=(12+4x)/(6)`

- Write
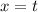`x=t` and substitute it into the equation above:

`y=(12+4t)/(6)`

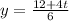
B) - Sove for
`x`:

`x=(6y-12)/(4)`

- When you substitute
`y=t` into the equation above, you obtain:

`x=(6t-12)/(4)`

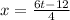
The answer is:
`x=t; y=(12+4t)/(6) \\ y=t; x=(6t-12)/(4)`