Question

HELP FASTTTT Which graph shows the solution to the system of linear inequalities ?

X - 4y is less than or equal to 4

Y < x + 1

Answer 1

First, look at the equation

`y < x + 1`
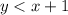
We can find the line

`y = x + 1`
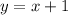
on a graph (drawing attached). It forms a diagonal line. Since y is less than, not equal, to x + 1, we shade the graph below that line.

Only one graph shows this correctly.

To find the graph for the equation

`x - 4y \leqslant 4`
we first have to simplify the equation:

`x - 4y \leqslant 4 \\ - 4y \leqslant 4 - x \\ y \geqslant - 1 + (1)/(4) x \\ y \geqslant (1)/(4) x - 1`
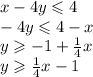
First, we subtract x from both sides.
Then we divide -4 from both sides, switching the direction of the inequality because we divided by a negative number.
We can now use this equation to find a graph.

The greater than or equal to sign means that we shade upward from the line (drawing attached).

`y = (1)/(4) x - 1`


As for which graph, it is graph #1, since the line for

`y < x + 1`
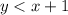
is a dotted line, not a solid line, because the < sign prevent the equation from fully reaching the line.

Answer 2

Well, we do not see the lines clearly (if they are dotted (inequality) or solid (inequality or equal) ), but we'll come back to this.

y<x+1 can be simplified to be y<x if we ignore the constants, so the valid part is BELOW the line, since (y<)

x-4y <=4
again, ignore the constants and simplify to
x-y<0 or
y>x so the valid part is ABOVE the line (since y>)

There are two graphs that satisfy BOTH conditions, pink (slope=1) at the bottom, and blue (slope = 1/4) on top, i.e. first and third graph.

For the absolute inequality (y<x+1), the red line must be dotted.
For the <= condition, the blue line must be solid.

As we cannot see the lines clearly, you will make the choice between the first and third graphs according to the previous paragraph.