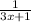Question

Simplify the expression given below. x+2/X^3+2x^2-9x-18 divided by 3x +1/x^2-9

Answer 1

Answer:


`(1)/(3x+1)`

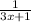Step-by-step explanation:

1) Expression given:


`( (x+2)/(x^3+2x^2-9x-18) )/( (3x+1)/(x^2-9) )`

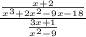
2) Factor the denominator of the first fraction:


`x^3+2x^2-9x-18=(x^3+2x^2)-(9x+18)=x^2(x+2)-9(x+2)=`


`(x+2)(x^2-9)`

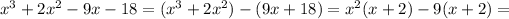
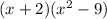
3) Rewrite the expression


`( (x+2)/((x+2)(x^2-9)) )/( (3x+1)/(x^2-9) )`

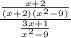
4) In the upper fraction x+2 in the numerator cancels out with x + 2 in the denominator.


`( (1)/(x^2-9) )/( (3x+1)/(x^2-9) )`

5) x² - 9 in both denominators cancel out each other, and the final result is:


`(1)/(3x+1)`