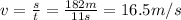a) The position of the particle along the straight-line path is given by
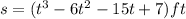
where t is the time.
If we substitute t=11 s inside the equation, we find the total distance covered during this time, in feet:
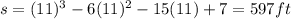
which converted into meters is s=182 m.
b) The average velocity of the particle at t=11 s is equal to the total distance travelled, s=597 ft, divided by the time taken, t=11 s:

We can also calculate it in meters/second: