Question

If an aqueous solution of urea n2h4co is 26% by mass and has density of 1.07 g/ml, calculate the molality of urea in the soln

Answer 1

`m=5.855m`

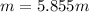
Step-by-step explanation:

Hello,

Molality is defined as:

`m=(n_(N_2H_4CO))/(m_(solvent))`

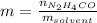
Thus, as the solution is % by mass, one assumes that the mass of urea is 26g and the mass of the solution 100g, in such a way, one computes the moles of urea by knowing its molecular mass:

`n_(N_2H_4CO)=26gN_2H_4CO*(1molN_2H_4CO)/(60gN_2H_4CO)=0.433molN_2H_4CO`

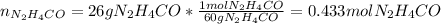
Now, as solution has a mass of 100g, the solvent has a mass of 74g which corresponds to 0.074L since the density of water is 1g/mL; in such a way the molality is:

`m=(0.433mol)/(0.074kg)=5.855m`

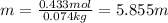
Best regards.

Answer 2

Answer is: molality of urea is 5.84 m.

If we use 100 mL of solution:
d(solution) = 1.07 g/mL.
m(solution) = 1.07 g/mL · 100 mL.
m(solution) = 107 g.
ω(N₂H₄CO) = 26% ÷ 100% = 0.26.
m(N₂H₄CO) = m(solution) · ω(N₂H₄CO).
m(N₂H₄CO) = 107 g · 0.26.
m(N₂H₄CO) = 27.82 g.
1) calculate amount of urea:
n(N₂H₄CO) = m(N₂H₄CO) ÷ M(N₂H₄CO).
n(N₂H₄CO) = 27.82 g ÷ 60.06 g/mol.
n(N₂H₄CO) = 0.463 mol; amount of substance.
2) calculate mass of water:
m(H₂O) = 107 g - 27.82 g.
m(H₂O) = 79.18 g ÷ 1000 g/kg.
m(H₂O) = 0.07918 kg.
3) calculate molality:
b = n(N₂H₄CO) ÷ m(H₂O).
b = 0.463 mol ÷ 0.07918 kg.
b = 5.84 mol/kg.