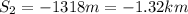Question

hapter 02, Problem 10 In reaching her destination, a backpacker walks with an average velocity of 1.15 m/s, due west. This average velocity results, because she hikes for 6.07 km with an average velocity of 2.78 m/s due west, turns around, and hikes with an average velocity of 0.675 m/s due east. How far east did she walk (in kilometers)?

Answer 1

The total average velocity
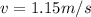
`v=1.15 m/s` (I assume west as positive direction) is given by the total displacement, S, divided by the total time taken, t:

`v= (S)/(t)=(S_1+S_2)/(t_1+t_2)`

where:
-The total displacement is the algebraic sum of the displacement in the first part of the motion (
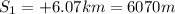
`S_1 = +6.07 km=6070 m`, due west) and of the displacement in the second part of the motion (
`S_2`, due east).
-The total time taken is the time taken for the first part of the motion,
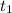
`t_1`, and the time taken for the second part of the motion,
`t_2`.
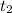
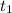
`t_1` can be found by using the average velocity and the displacement of the first part:

`t_1 = (S_1)/(v_1)= (6070 m)/(2.78 m/s)=2183 s`

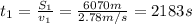
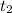
`t_2`, instead, can be written as
`t_2= (S_2)/(v_2)`, where
`v_2=-0.675 m/s` is the average velocity of the second part of the motion (with a negative sign, since it is due east).
Therefore, we can rewrite the initial equation as:

`v= 1.15 =(6070+S_2)/(2183- (S_2)/(0.675) )`
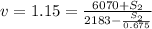
And by solving it, we find the displacement in the second part of the motion (i.e. how far did the backpacker move east):

`S_2 = -1318 m=-1.32 km`