Question

What is the equation in slope-intercept form of the line that passes through the point (12, 5) and is perpendicular to the line represented by y=34x−8?

Answer 1

The equation in slope-intercept form of the line that passes through the point (12, 5) and is perpendicular to the line is:

• 
  `y=-(1)/(34)x+(91)/(17)`

Explanation:

We know the slope-intercept form of the line equation

`y=mx+b`

where m is the slope and b is the y-intercept

Given the line

`y=34x-8`

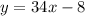
comparing with the slope-intercept form of the line equation

The slope m = 34

We know that a line perpendicular to another line contains a slope that is the negative reciprocal of the slope of the other line, such as:

slope = m = 34

Thus, the slope of the the new perpendicular line = – 1/m = -1/34 = -1/34

Using the point-slope form

`y-y_1=m\left(x-x_1\right)`

where m is the slope of the line and (x₁, y₁) is the point

substituting the values of slope = -1/35 and the point (12, 5)

`y-y_1=m\left(x-x_1\right)`

`y-5=-(1)/(34)\left(x-12\right)`

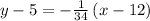
Add 5 to both sides

`y-5+5=-(1)/(34)\left(x-12\right)+5`

`y=-(1)/(34)x+(91)/(17)`

Therefore, the equation in slope-intercept form of the line that passes through the point (12, 5) and is perpendicular to the line is:

• 
  `y=-(1)/(34)x+(91)/(17)`