Question

What are the answers to these 2 question 4&5 PLZ HELP

Answer 1

The first problem:

y = -2 x² ⇒⇒⇒ x² = -y/2 →(1)33 x² + y² = 27 → (2)
By substitution from (1) at (2) with the value of x²
∴ 33 (-y/2) + y² = 27
∴ y² - 16.5 y - 27 = 0
a = 1 , b = -16.5 , c = -27
∴
`y= (-b \pm √(b^2-4ac) )/(2a) = (16.5 \pm √((-16.5)^2-4*1*(-27)) )/(2*1)`
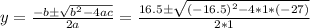
∴ y = 18 or y = -3/2
By substitution from at (1) with the value of y
for y = 18 ⇒⇒⇒ x² = -18/2 = -9 (unacceptable)
for y = -3/2 ⇒⇒⇒ x² = -(-3/2)/2 = 3/4
∴
`x= \pm \sqrt{ (3)/(4) } = \pm ( √(3) )/(2)`
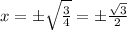
The correct options are 2 , 7
Solution of the system of equations is

`( ( √(3) )/(2) , (-3)/(2) )`
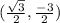
and

`( -(√(3) )/(2) , (-3)/(2) )`
==================================
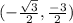
The second problem:
The general equation of the hyperbole is

`(x^2)/(a^2) - (y^2)/(b^2) =1 Transverse axis is horizontal The equation if the asymptotes are y = \pm (b)/(a)x`
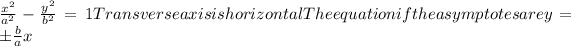
For the given equation:

`(x^2)/(225) - (y^2)/(36) = 1`
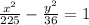
a² = 225 ⇒⇒⇒ a = √225 = 15
b² = 36 ⇒⇒⇒ b = √36 = 6
∴ the slope of the asymptotes = b/a and -b/a

b/a = 6/15 = 2/5

-b/a = -6/15 = -2/5

∴ m = 2/5 and m = -2/5