Question

​ G=−3w 4 +2w 2 z 2 +5z 4 P=8w 4 −3w 2 z 2 +z 4 ​ P-G=P−G=P, minus, G, equals Your answer should be a polynomial in standard form.

Answer 1

Please take the time to type in the question by yourself. I assume you use some kind of an apps to transcribe problems. It only confuses and discourages helpers. The fact that the question was reported means it is not clear.

I ASSUME the question is as follows:

`G=-3w^4+2w^2z^2+5z^4`

`P=  8w^4-3w^2z^2+z^4`
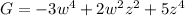
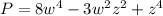
Need to find P-G.

We see clearly that each expression P, G contains 3 terms, namely w^4, w^2z^2 and z^4. We will have to add/subtract like terms to get the answer.


`P-G=8w^4-3w^2z^2+z^4-(-3w^4+2w^2z^2+5z^4)`

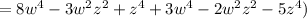
`=8w^4-3w^2z^2+z^4+3w^4-2w^2z^2-5z^4)` (distribute negative sign into expression G)

`=8w^4+3w^4-3w^2z^2-2w^2z^2+z^4-5z^4` (group like terms)

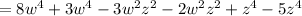
`=11w^4-5w^2z^2-4z^4` (add/subtract like terms)

Answer 2

To find the solution to this question, we need to perform a subtraction operation between the two given polynomials, P and G.

The given polynomials are:

G = -3w^4 + 2w^2z^2 + 5z^4,

and

P = 8w^4 - 3w^2z^2 + z^4.

First, let's line up the like terms of both polynomials, which means organizing the terms of both polynomials according to the power they are raised to:

G = -3w^4 + 2w^2z^2 + 5z^4,

P = 8w^4 - 3w^2z^2 + z^4.

We will be carrying out P - G (subtract G from P). Subtraction involves changing the signs of each term in polynomial G and then adding it to polynomial P:

Result = P - G = 8w^4 - (-3w^4) - 3w^2z^2 - (2w^2z^2) + z^4 - (5z^4)
This then simplifies to,

Result = 8w^4 + 3w^4 - 3w^2z^2 - 2w^2z^2 + z^4 - 5z^4
Result = 11w^4 - 5w^2z^2 - 4z^4.

This is a polynomial in standard form that uses the difference between two other polynomials.